Chap 14 Review

$$$$

Loading webR...

Figure 1: .

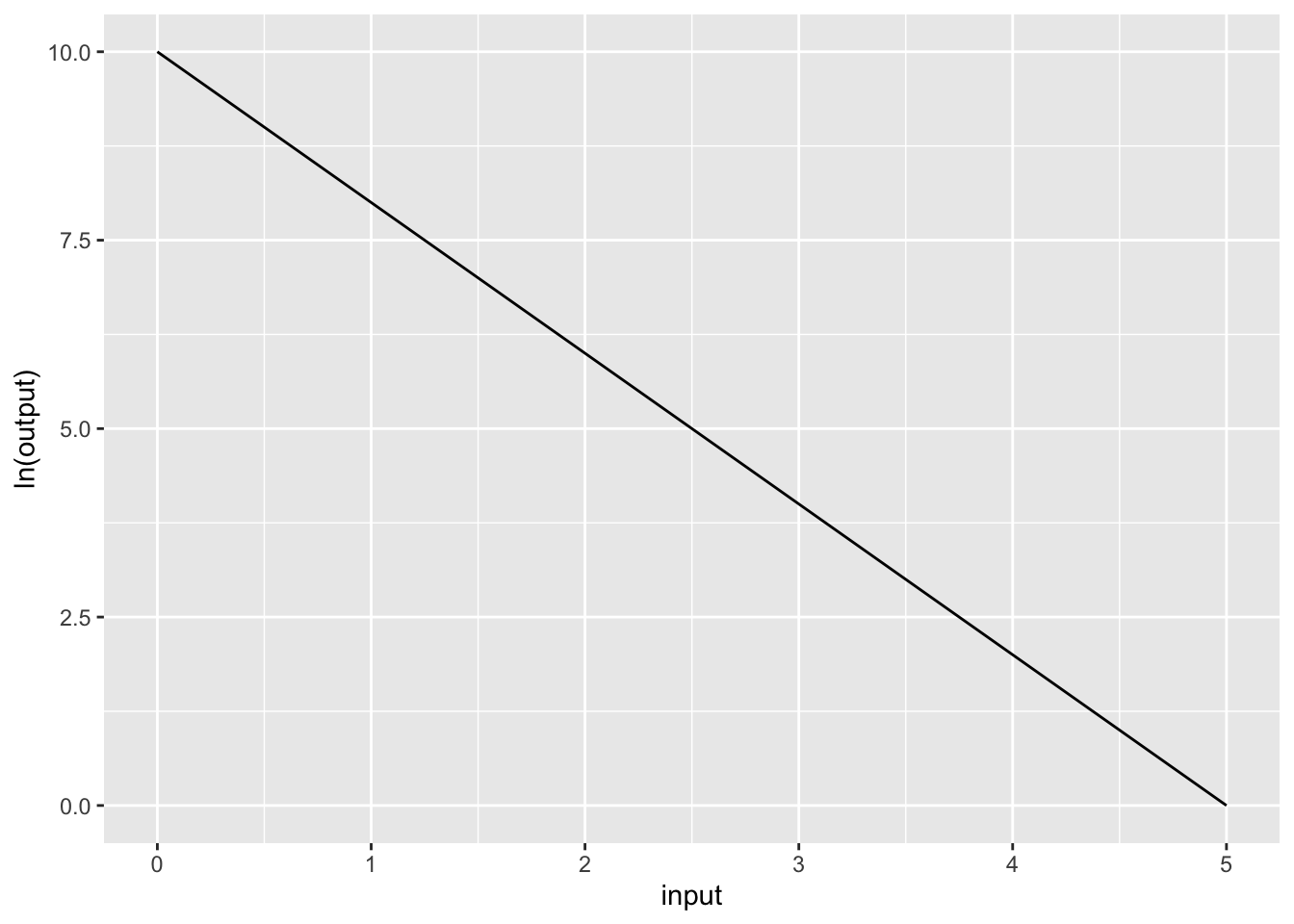
Exercise 1 What is the correct form for the relationship shown in Figure 1

$g(x)\equiv e^{10}{e}^{-2x}$

$g(x)\equiv 10e^{-2x}$

$g(x)\equiv e^{10}{e}^{-1.5x}$

$g(x)\equiv e^{-1.5}{e}^{10x}$

$g(x)\equiv 10x^{-1.5}$

question id: drill-Scales-1

Exercise 2 Which of these is the better modeling relationship for the following scenario: Your data looks linear on a semi-log plot.

Power-law       Exponential       Logarithmic      

question id: drill-Scales-1b

Exercise 3 Which of these is the better modeling relationship for the following scenario: Your data looks linear on a log-log plot.

Power-law       Exponential       Logarithmic      

question id: drill-Scales-1c

Figure 2: .

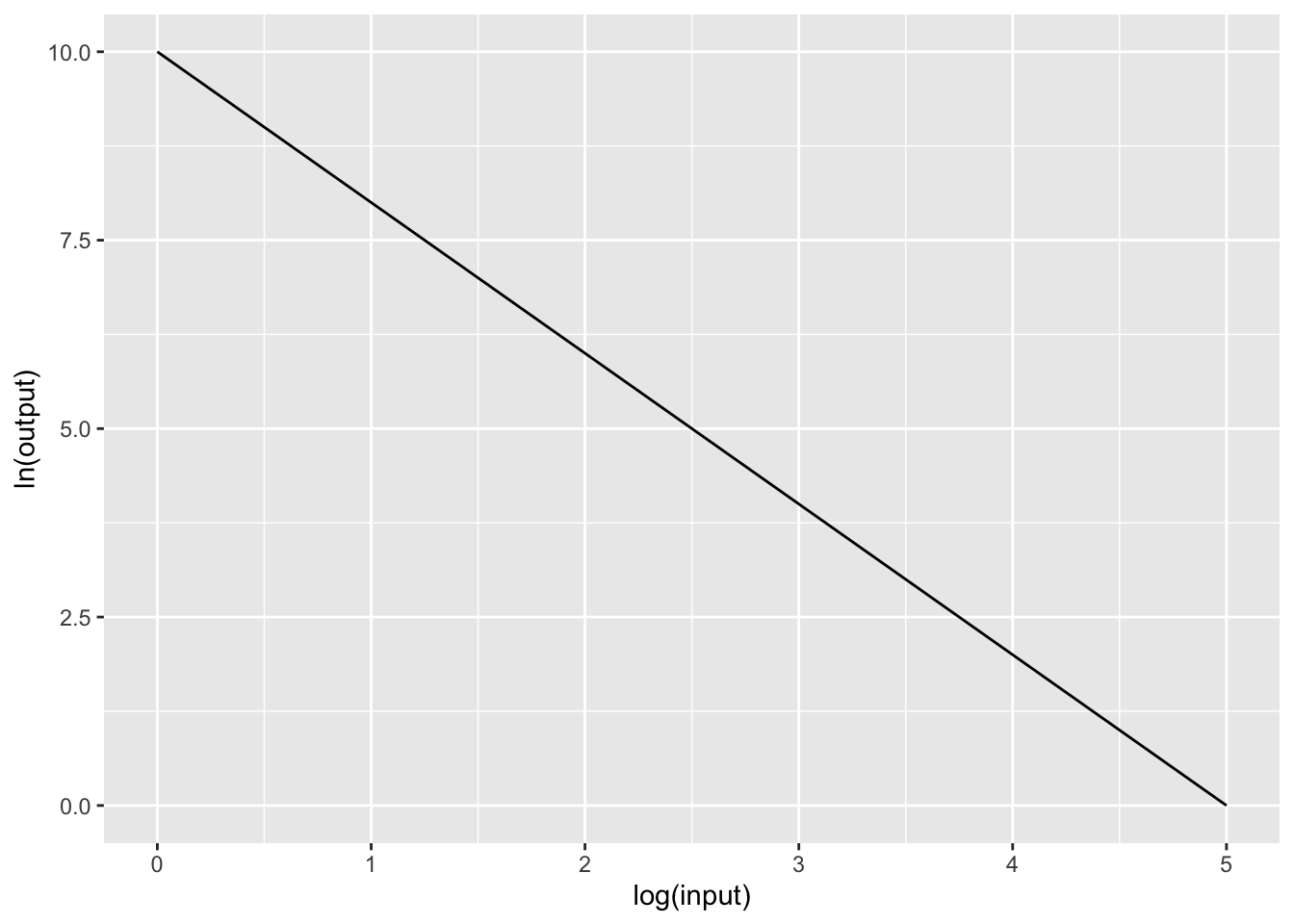
Exercise 4 What is the correct form for the relationship shown in Figure 2?

$g(x)\equiv 10x^{-2}$

$g(x)\equiv e^{10}{e}^{-1.5x}$

$g(x)\equiv e^{10}{x}^{-2}$

$g(x)\equiv e^{1}0x^{-1.5}$

question id: drill-Scales-2

Figure 3: .

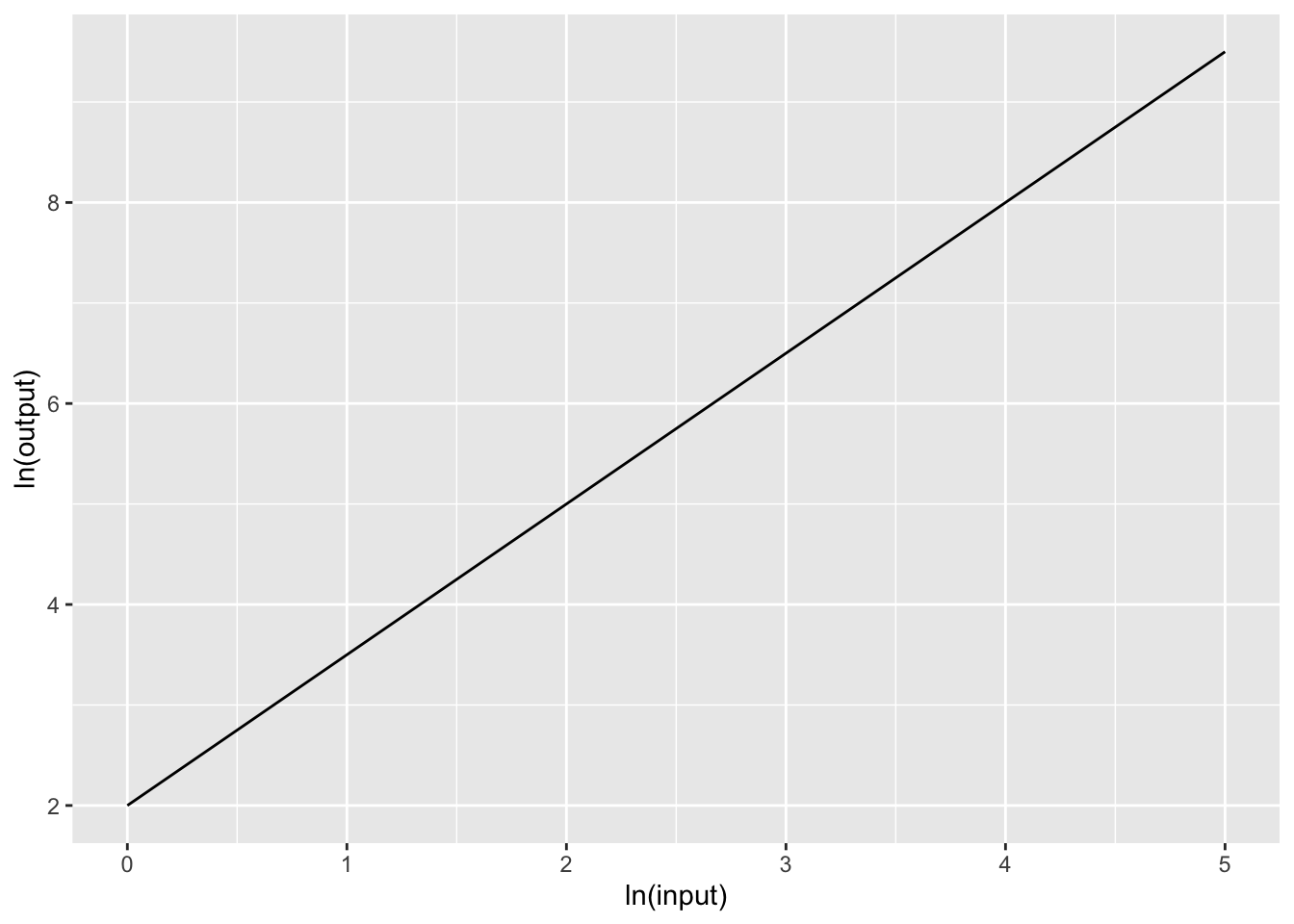
Exercise 5 What is the correct form for the relationship shown in Figure 3?

$g(x)\equiv e^2{e}^{1.5x}$

$g(x)\equiv 2x^{1.5}$

$g(x)\equiv e^2{x}^{1.5}$

$g(x)\equiv e^2{x}^2$

$g(x)\equiv e^2{x}^{-1.5}$

question id: drill-Scales-3

Figure 4: .

Exercise 6 Figure 4 shows a horizontal axis for a graph. How can you tell that this is a logarithmic axis?

The labels are all multiples of 2.

The labels are evenly spaced and each label is a factor of 2 larger than the previous one.

Trick question. It is not a log scale.

question id: drill-Scales-4

Figure 5: .

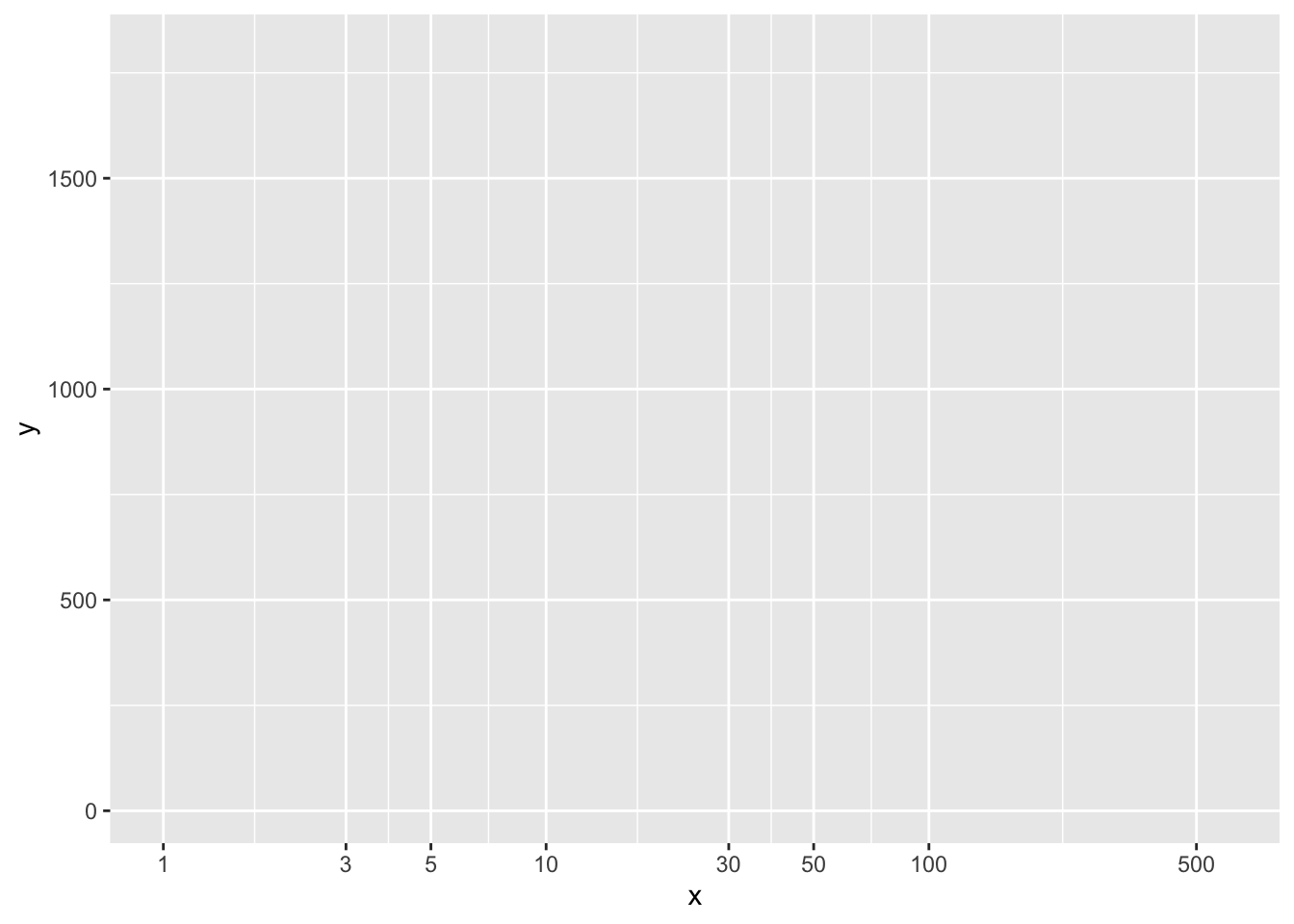
Exercise 7 Figure 5 shows a horizontal axis for a graph. How can you tell that this is a logarithmic axis?

The labels are 1, 3, 5, 10, …

The labels are evenly spaced and each label is a factor of 3 larger than the previous one.

The 3 label is about halfway between the 1 and 10 label for each decade, and the 1 and 10 labels have the same spacing for every decade.

Trick question. It is not a log scale.

question id: drill-Scales-5

Figure 6: .

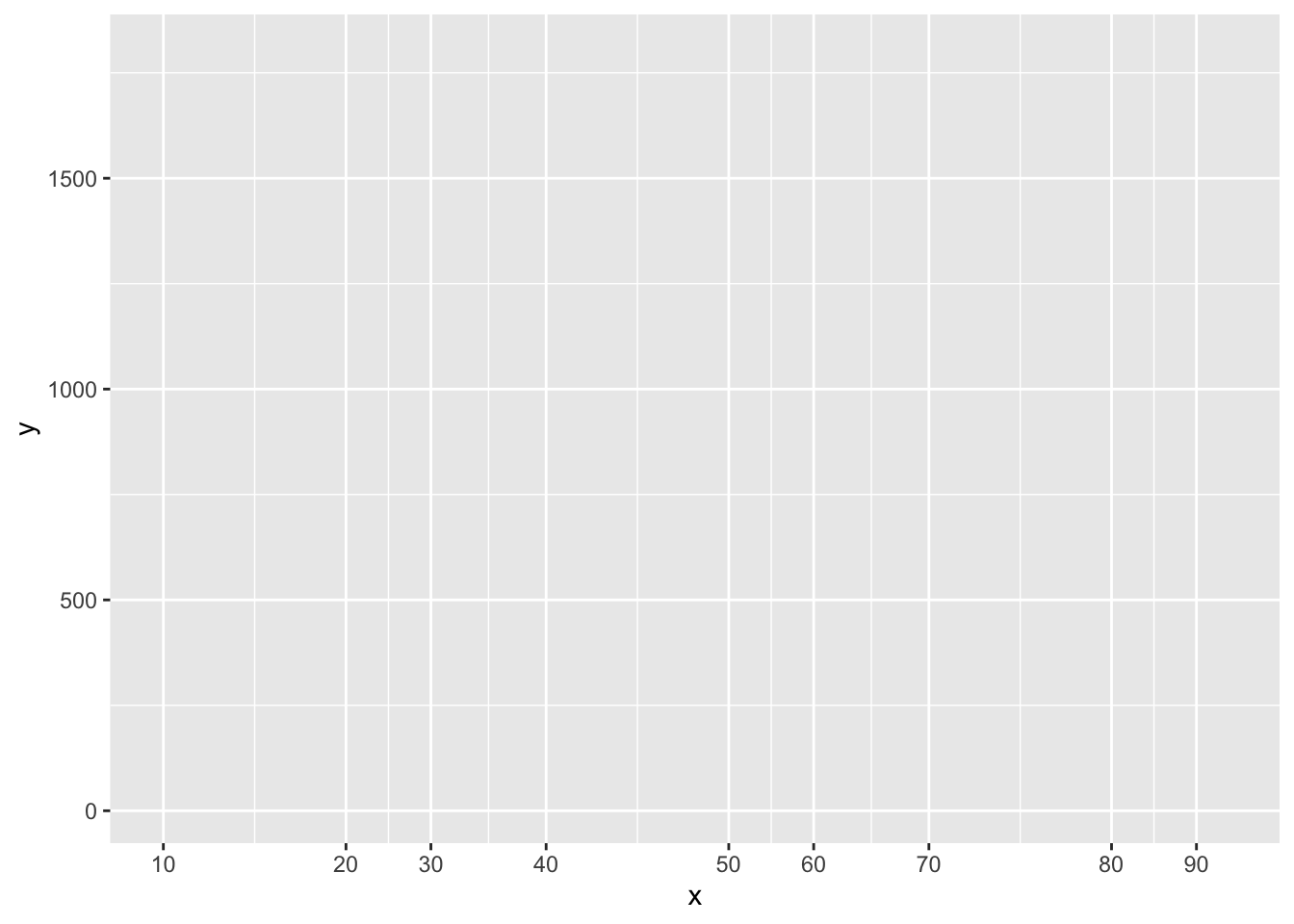
Exercise 8 Figure 6 shows a horizontal axis for a graph. How can you tell that this is a logarithmic axis?

The labels are 10, 20, 30, 40, …

Each label is 10 + the previous label.

The 3 label is about halfway between the 1 and 10 label for each decade, and the 1 and 10 labels have the same spacing for every decade.

Trick question. It is not a linear scale.

question id: drill-Scales-6

14-review.rmarkdown

No answers yet collected