6  Describing functions

$$$$

Loading webR...

Reading questions

Reading question 6.1 Which of these is not a function-shape concept discussed in Chapter 6?

periodicity       concavity       curl       monotonicity      

question id: 6-words

Reading question 6.2 Draw a graph of a periodic function of your own construction. Use your imagination. (Bring your drawing to class, either as a phone/picture or as a piece of paper.)

For grading purposes, once you have made your drawing, copy the following into the text box:

I have honorably completed the periodic-function drawing task.

Reading question 6.3 Which of these pattern-book functions are monotonic?

reciprocal       square       sigmoid       gaussian      

question id: monotonic-fun

Reading question 6.4 Does the exponential function have a local maximum?

yes       no      

question id: exp-max

Reading question 6.5  

Figure 6.1: Reproduced from Chap 6 of MOSAIC Calculus

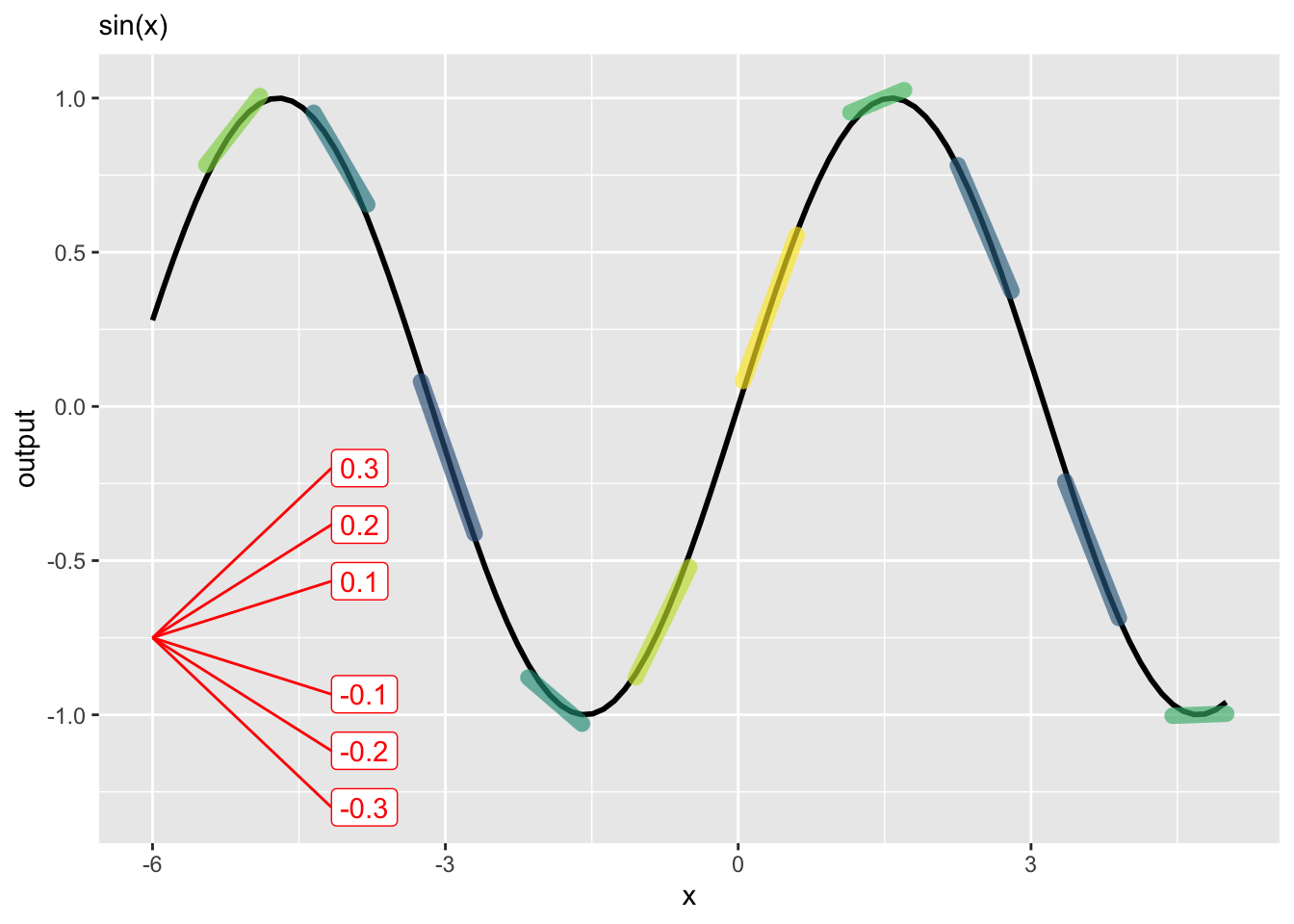
The following questions refer to Figure 6.1.

1. At which of these value of $x$ does the function have a negative slope?

-5       -3       0       1      

question id: sin-slope-a

2. The red annotations in Figure 6.1 indicate the numerical value of lines drawn at the indicated slope. Which of these values of $x$ is closest to an input value where the function has a slope of about 0.3?

-4.5       -0.2       1.7       4.0      

question id: mc-sin-slope-b

3. Again referring to the red annotations in Figure 6.1 … The line labelled 0.3 seems to be at about 45 degrees to the horizontal. This corresponds to a slope value of 1. Yet the line is marked as having a slope of 0.3, an apparent paradox. Give an explanation that accounts for the paradox.

06-reading.rmarkdown

No answers yet collected